So, I have had some calls to expand on the theme of my Gamma
Trap post, written a little while ago. Read it here,
if you haven't already. In fact, maybe
everyone should go back and read that to get started, because it will be
helpful to have your Option Geek hat firmly on your head before you dive
into this concept: Vega.
Before we dig deeply into the Greeks (and a wee bit of
math), let me first step back and say why the Vega insight is important. Vega is
ultimately the route by which professional option traders make money. It's that
simple. Our good friends over at the FHLB, the FFCB, and FNMA all know this
game very well, as do option professionals. By no means am I urging you to become
an active option trader, but you are already playing in this wonderful game quite
often, whether you like it or not. Most readers of this article are generally
short options throughout their balance sheets—hopefully knowingly. Where? In
prepayment risk or extension risk in callable bonds, loans, deposits, advances…and
the list goes on.
So, what is this Vega of which I speak? Vega represents volatility
or, more precisely, the implied
volatility. This magical input is what ultimately drives the price of an
option. It is important to understand that professional option traders
generally "make" market prices (input the implied volatility levels) and the
public generally "takes" market prices. To wit, when I monitor callable bond
issuance, this is what I see:
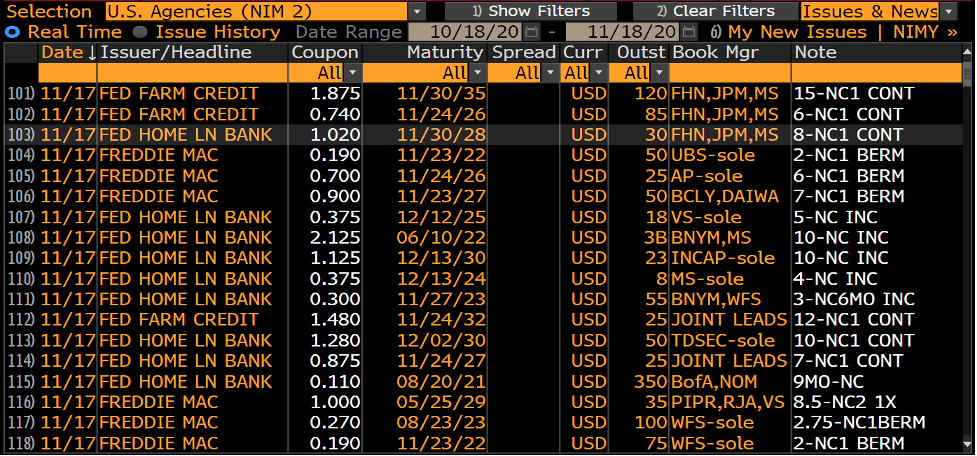
This is the New Issue Monitor via our good friends at
Bloomberg. It posts all of the new issuance across the market, all day, every
day. Most people will only see selected offerings from this daily onslaught.
Taker vs. Maker
What follows will be a tricky, but very important,
differentiator for you. I have to admit that it is not easy to explain, but I
will do my best.
Option pricing was revolutionized when firms at the Chicago
Board of Trade and other exchanges actually started applying the Black-Scholes
model to trading options on a real-time basis. The most notable of these firms
was CRT (Chicago Research and Trading). During a significant portion of the
1980s, CRT was executing more option trades than any other firm in the world.
The founder of the firm, Joe Ritchie, famously said:
"A
trader on the floor with the simplest programming calculators in 1976 instantly
became a one-eyed man in the land of the blind."
The secret sauce to this all was an understanding of Vega.
The Black Scholes model is not all that difficult. To solve for
the price of an option, you need to know the price of the underlying asset, the
strike price, the risk-free interest rate, and the time to maturity. Oh, and
you also need to know the price volatility of the asset. In other words,
its Vega. Of these inputs, all are knowable—and the same for everyone—except
Vega. Future volatility is unknown, and unknowable. We can know the historic volatility of an asset (or
group of assets), but we have to make an assumption to input Vega into a
black-Scholes model. Once all of these inputs are established, then we can
solve for the price of the option.
Prior to CRT (and a couple others), option prices were kind of
negotiated and effectively guessed. Truly.CRT became the first real market maker in the options pits. They could,
would, and did make a market in any option, at any time, with conviction and with
accuracy. They were the price maker, while (most) everyone else was a price taker.
And so, here we are again—makers vs. takers. When someone buys a 7-year
non-call 1 at 0.90% (see above), the loop is completed. I can know all of the
inputs into the equation. The price of the option has been set at 0.90% minus
the yield of the underlying. In this case, a bullet with the same maturity date
would have yielded approximately 0.75%. So, the option has been taken by the
market at 0.15%. A professional trader would be quickly putting this
information into the Black-Scholes model and solving to "back out" what implied
volatility corresponds to this pricing. We can actually get a glimpse of
approximately where these realized volatilities have been via the MOVE index. In
the graphic, I've inserted a horizontal line to show the average for the last two
years at a level of 62.1206.
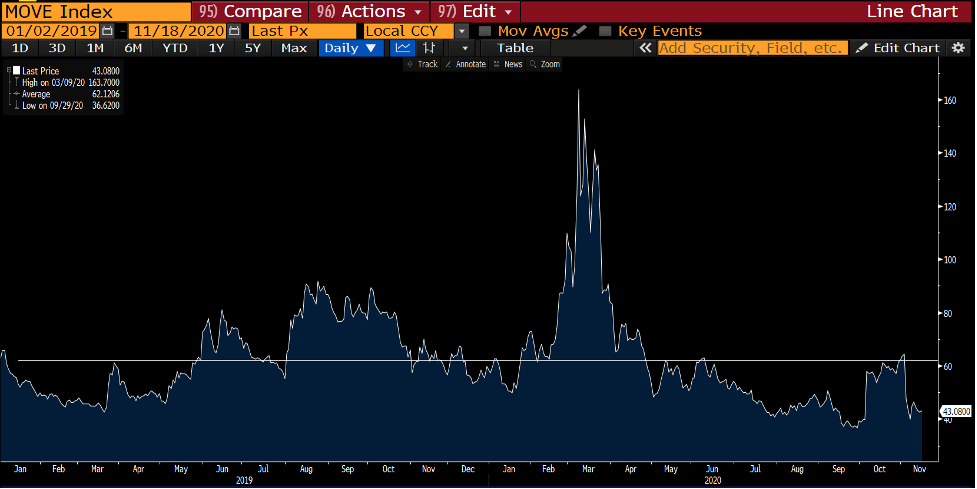
Ok, so I've had you swimming in the deep end of the pool for a
while, what's my point?
Back to my title: If the Gamma doesn't get you, the Vega just might.
I think most people are familiar with the simple rule of thumb: yields up means
prices down, and yields down means prices up. Most of the time, we think of
this in terms of the actual treasury yield curve moving, but the hidden player can
actually be the Vega.

Let us consider the aforementioned 7-year non-call 1 at 0.90%. If
implied volatilities surge (or just go up), then the option price will
increase. That is a fact. And so, without the underlying bullet's yield moving
at all, the price of the callable bond will go down. The makers will use the
Black-Scholes model once again, input a new, higher value for implied
volatility, and they will set a lower price for the callable bond.
This is particularly troublesome if you a consistently short
options (like most readers here), because suddenly everything is upside down. All of your asset prices will drop as
the makers start resetting prices. This of course will eventually lead to the
Gamma trap from the earlier post.
Those darn Greeks! Some of you may be thinking—who cares? You're not
a professional options trader, so why should you spend time thinking about
implied volatilities? You most likely have never thoroughly considered the
Black-Scholes model as a daily tool in the market place that can affect your
balance sheet valuations from loans to wholesale fundings. Most of you should
not be spending too much energy
thinking about that! But you are
competing in a high stakes game. Even if you don't consider yourself a
professional options trader, you are a professional, and you do trade options
implicitly all the time. Unfortunately, you are usually in the position of taker,
not maker, the exception being in your ability to "make" the prices for the
options you allow in your retail products, like loan prepayability and early CD
surrender penalties.
But in general, you are a taker, which is actually okay, as long
as you understand the forces at play.
Among other reasons, you have edges that the pure option trader often
does not. For example, you can decide to avoid playing in options at all in
some places, such as in your bond portfolio or in your advance stack when the makers
aren't paying you enough to take them (i.e., they are setting Vega so low as to
make these options unrewarding). Understanding
the maker's game and the impact of broader options pricing can really give you
an advantage in your game, the one we
call investing/banking.
Final, final thought: Christmas
is only 37 days away and I hear some of the shipping companies will not be able
to handle all of the AMZN orders. Get ahead of the curve, or you may experience
some serious Christmas Morning Vega!
Be sure to fill out the form below to subscribe to my weekly blog.


